Mandelbrot’s got our number

Originally published 27 July 1992

For the jack­et of my col­lec­tion of sci­ence essays, the pub­lish­er pro­posed a rep­re­sen­ta­tion of the Man­del­brot set, a com­put­er-gen­er­at­ed math­e­mat­i­cal pat­tern of won­der­ful com­plex­i­ty, one of a fam­i­ly of pat­terns called frac­tals.

“But there’s not a sin­gle word about frac­tals in this book,” I protested.

“Nev­er mind,” he said. “It’s the hottest graph­ic image in sci­ence today.”

The hottest graph­ic image? What’s going on here? Are sci­ence essays a pop com­mod­i­ty, to be pack­aged like a rock video? Is math­e­mat­ics now a mat­ter of fashion?

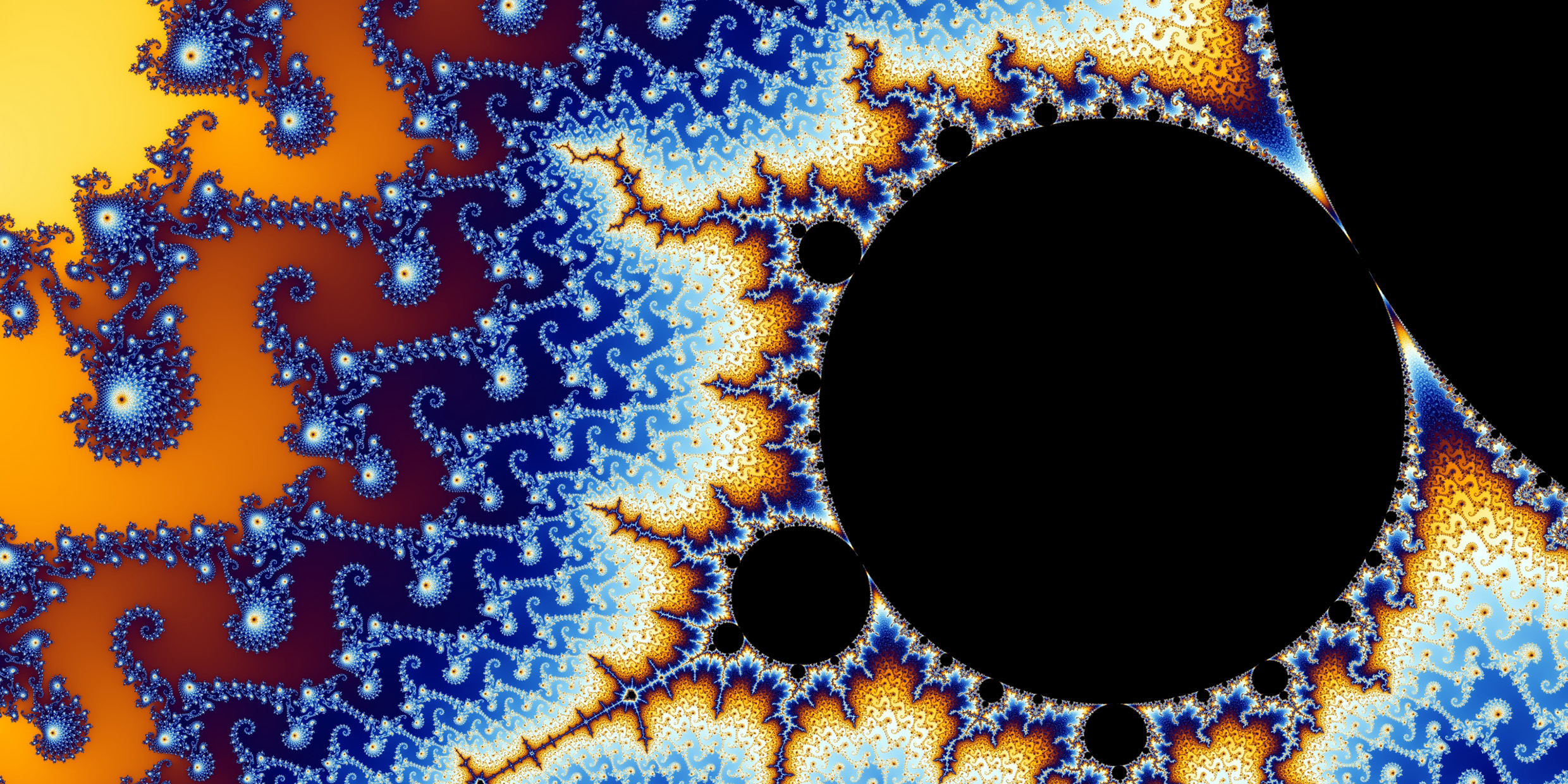
You bet! And noth­ing is more fash­ion­able than the Man­del­brot set.

It has been called the most com­pli­cat­ed object in math­e­mat­ics, but in anoth­er sense, it is exceed­ing­ly sim­ple. It is cer­tain­ly one of the most beau­ti­ful objects in math­e­mat­ics, and one of the most philo­soph­i­cal­ly provoca­tive. It was dis­cov­ered in 1979 by the icon­o­clas­tic genius Benoit Man­del­brot.

Bear with me on the next para­graph; there is light at the end of the tunnel.

The Man­del­brot set is the col­lec­tion of all com­plex num­bers that behave in a cer­tain way. A com­plex num­ber has a real and an imag­i­nary part (the imag­i­nary part is a real num­ber mul­ti­plied by the square root of minus one). Here’s how to decide if a num­ber is in the set: Take a com­plex num­ber, square it, add the orig­i­nal num­ber, square the result, add the orig­i­nal num­ber again, square the result, and so on, over and over; if the result remains finite (does­n’t become infi­nite­ly large), then the orig­i­nal num­ber is in the Man­del­brot set; if the result becomes infi­nite­ly large, then the orig­i­nal num­ber does­n’t qualify.

What could be sim­pler than that? This is the kind of cal­cu­la­tion that needs a com­put­er. In fact, the Man­del­brot set could nev­er have revealed its won­ders until high-speed com­put­ers came along.

When the num­bers in the Man­del­brot set are plot­ted on a two-dimen­sion­al graph, extra­or­di­nary pat­terns emerge — swirls, flour­ish­es, and fil­i­grees of lux­u­ri­ous com­plex­i­ty. When these are dis­played in col­or on a com­put­er screen they are exquis­ite­ly beautiful.

But can beau­ty alone explain why these pat­terns have become a pop phe­nom­e­na? Why do peo­ple with no inter­est in math­e­mat­ics go ga-ga over frac­tals? The answer, my friends, is blow­ing in the wind.

Lit­er­al­ly.

No oth­er kind of math­e­mat­ics so per­fect­ly mim­ics the antics of the wind as frac­tals. In the details of the Man­del­brot set are eddies, streams, and vor­tices. Here are big whorls with lit­tle whorls upon them, and yet small­er whorls upon those — glob­al cir­cu­la­tion pat­terns, hur­ri­canes, whirl­winds, dust dev­ils. Deep­er inspec­tion of the set yields ever more details — small­er pat­terns sim­i­lar to the larg­er pat­terns, and yet dif­fer­ent, reced­ing into infin­i­ty, like those mar­velous pho­tographs of cyclones in the atmos­phere of Jupiter sent back by the Voy­ager spacecraft.

And it is not just the winds that frac­tals resem­ble, but tum­bling brooks, flow­ers, ferns, leafy trees, and the cir­cu­la­tion of the blood. It is the organic­i­ty of the Man­del­brot set that accounts for its pop­u­lar­i­ty, the way in which it com­bines sim­plic­i­ty with infi­nite vari­a­tion. The Man­del­brot set taps into a fun­da­men­tal human ambiva­lence — our desire for order and our fear of fixity.

Sci­ence has been won­der­ful­ly suc­cess­ful at sat­is­fy­ing our need for order. It has sought to reduce all of nature to a few fixed, invari­able laws. Its method is to know by iso­la­tion, by reduc­tion to unseen par­tic­u­lars. The fun­da­men­tal metaphor of sci­ence is the lab bench — a stark, clean, imper­me­able sur­face upon which one part of the world can be dis­con­nect­ed from all the rest.

But some­thing in our spir­it rebels against too much reduc­tion­ism. We are con­vinced in our heart of hearts that the uni­verse is an organ­ic uni­ty, and, as Goethe said, that dis­sec­tion destroys the very thing we wish to know. We thrive on the sta­bil­i­ty of law, but we rel­ish spon­tane­ity and sur­prise. Thus, our cul­ture’s love-hate rela­tion­ship with science.

The Man­del­brot set is a pow­er­ful pub­lic metaphor for a new kind of sci­ence, at once math­e­mat­i­cal and organ­ic. It remains to be seen whether the explana­to­ry pow­er of frac­tals can replace the sort of sci­ence we have enjoyed since New­ton. Cer­tain­ly, frac­tals have their prophets and cham­pi­ons, but so far the hoopla and pub­lic enthu­si­asm has out­stripped sol­id sci­en­tif­ic achievement.

Frac­tals, such as the Man­del­brot set, make won­der­ful com­put­er games; it’s less clear that these pret­ty pat­terns can replace the sci­ence that has served us so well for 300 years.

In the intro­duc­tion to his text­book on frac­tal math­e­mat­ics, Michael Barns­ley says, “[Frac­tals] will make you see every­thing dif­fer­ent­ly. There is a dan­ger in read­ing fur­ther. You risk loss of your child­hood vision of clouds, forests, galax­ies, leaves, feath­ers, flow­ers, rocks, moun­tains, tor­rents of water, car­pets, bricks, and much else besides.”

He’s got the right idea, but he stat­ed it back­wards. What is so pop­u­lar­ly appeal­ing about frac­tals is that they recap­ture some­thing of our child­hood vision of a world that is play­ful, visu­al­ly appeal­ing, and organ­i­cal­ly whole, pre­cise­ly the attrib­ut­es we do not find in the sci­ence we learned in school.